Cone Formulas for Competitive Exams
What is Cone?
A cone is a three-dimensional figure in
geometry that narrows smoothly from a flat base (usually circular base) to a
point(which forms an axis to the centre of the base) called the apex or vertex.
The real-life example is a birthday cap
in the shape of a cone. We can also define the cone as a pyramid which has a
circular cross-section, unlike pyramid which has a triangular cross-section.
These cones are also stated as a circular cone.
Types of Cone
As we have already discussed a brief definition of the cone, let’s talk about its types now. Basically, there are two types of cones;
- Right Circular Cone
- Oblique Cone
Right Circular Cone
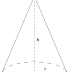
A cone which has a circular base and the axis from the vertex of the cone towards the base passes through the center of the circular base. The vertex of the cone lies just above the center of the circular base. The word “right” is used here because the axis forms a right angle with the base of the cone or is perpendicular to the base. This is the most common types of cones which are used in geometry. See the figure below which is an example of a right circular cone.
Oblique Cone
A cone which has a circular base but the axis of the cone is not perpendicular with the base, is called an Oblique cone. The vertex of this cone is not located directly above the centre of the circular base. Therefore, this cone looks like a slanted cone or tilted cone.
Cone Formulas
1. Cone surface area = Ï€r²+Ï€rl
or
surface area of a cone = πr (l + r)
2. Slant Height, l = √(r2+h2)
3. Volume of the Cone, Volume(V)
= ⅓ Ï€r2h
cubic units
Reviewed by SSC NOTES
on
July 07, 2023
Rating: